Yesterday I posted a review of a riflescope that had MIL dot markings on the reticle. In the comments there was some confusion about the purpose MIL dots have in range estimation, and so I wanted to break the answer out into a more complete article. It involves some math and a slight chance of high school flashbacks, but I swear it’s not that complicated. Let’s begin at the beginning, then…

Range estimation is useful in shooting when you’re not on a flat “known distance” range and need to hit something with a well aimed shot. Known distance ranges typically have markers you can use to determine how far away they are (MCB Quantico’s 1,000 meter known distance ranges have berms every 100-200 meters) but when those aides are absent you need to rely on other clues to know how far the bullet will drop before it hits the target and adjust your aim accordingly. One method of range estimation (preferred by most shooters) is by using MIL dots.

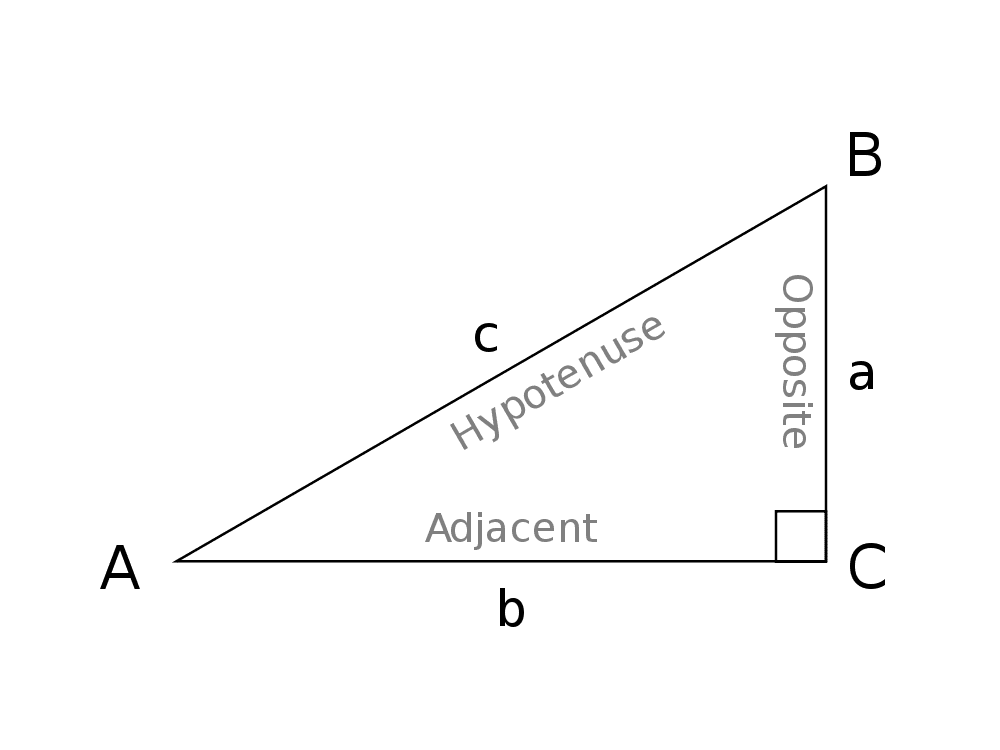
The theory behind MIL range estimation is basic trigonometry. If we assume that the target makes a right angle with the ground (standing up straight, angle “C”) and we estimate the height of the target (the average American male is 5 feet 9.5 inches, leg “a”) then the angle between the bottom of the target and the top of the target (from our viewpoint, angle “A”) can tell us how far away they are (leg “b”). The ratio of leg “a” to leg “b” is the tangent of angle “A” (SOH – CAH – TOA), and once we know the ratio it’s simple math to figure out the approximate number for leg “b”.

MIL dots provide a fixed reference on the scope that enables us to determine the angle between the top and bottom of the target. Fixed, that is, if they’re in the “first focal plane” of the scope. I talk about focal planes in another Ask Foghorn post but the basics are that first focal plane reticles will remain a constant size relative to a fixed target no matter the magnification. If the reticle is in the second focal plane the ratio between the target and reticle will change and make the markings basically useless. Second focal plane scopes typically let you know at what magnification the dots will be the “proper” size compared to the target but that’s one more thing you need to remember and one more thing to go wrong.

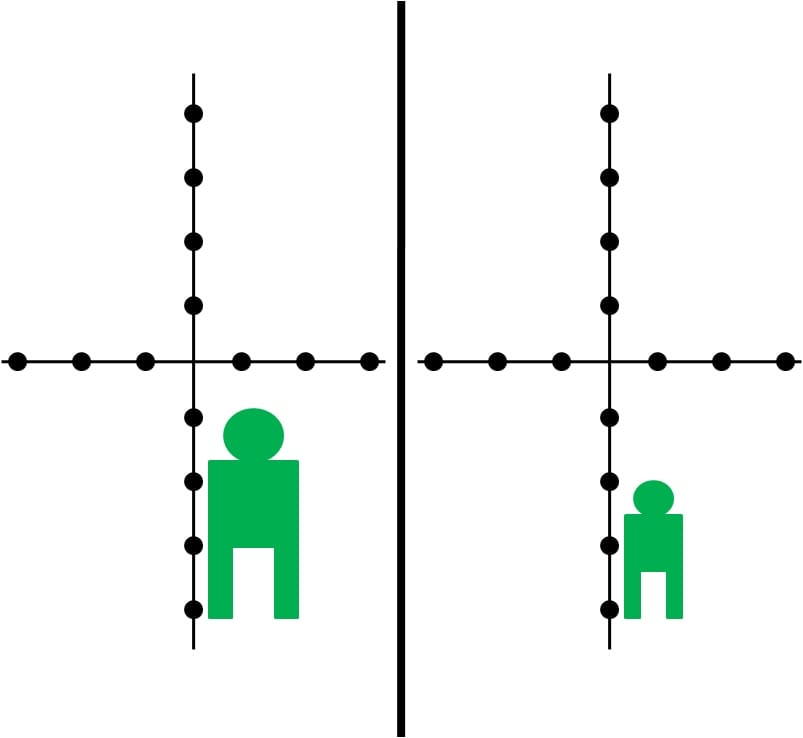
Thanks to the wonders of physics we know that an object appears to be “bigger” when we are closer to it and “smaller” when we are farther away from it. Objects appear to grow and shrink linearly, so a target twice as far away will be half as big. So if we assume that the badly drawn green man in the crosshairs above remains a constant size then the one on the left would be “closer” than the one on the right assuming the crosshairs are the same size (first focal plane or optics without variable zoom). If the reticle was in the second focal plane then this could be a target at the same distance but just with a different zoom setting. But for now let’s continue with the first case.

MIL dots allow us to dispense with the complicated mathematics of trigonometry, as the “simple” formula has been derived for us thanks to the standard distance between MIL dots. Here it is:

This equation (simplified from the Wikipedia equation for distance in imperial units) gives us the distance in yards to a target whose actual size (in inches) we know and whose apparent size (in MILs) we can measure through our scope, where “H” is the height in inches, “M” is the apparent height in MILs, and “D” is the distance to the target in yards.

For our example poorly drawn human in the left hand reticle, assuming they are a standard American male (69.5 inches) and measuring them at about 3 MILs high in the scope they would appear to be 643.57 yards downrange. For the right hand reticle (smaller poorly drawn man) they would calculate out to be 965.36 yards downrange — the target is 1/3 smaller and therefore 1/3 further away from us.

And that’s how range estimation using MIL dots works. If any of this is unclear let me know and I’ll do my best to make it easier to understand.

If you have a topic you want to see covered in a future “Ask Foghorn” segment, email [email protected].

To browse previous Ask Foghorn segments visit http://www.thetruthaboutguns.com/category/askfoghorn/.

RELATED ARTICLESMORE FROM AUTHOR

28 COMMENTS

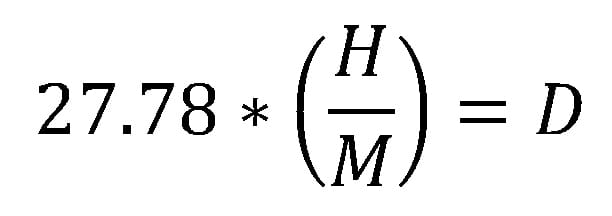
  1. “… the average American male is 5 feet 4 inches…”

    That can’t be right. I’m 5′ 4″ so I speak from experience of always being the shortest guy in the room… 😉

    Maybe if you include children.

  2. I would really like a comprehensive list of scopes that are first focal plane. Maybe a buyer’s guide? Including some fixed power scopes?

    I had a bear of a time trying to find one yesterday.

  5. All of this nonsense about First focal plane vs Second is all personal bias. Learn your equipment, know your magnification plain and simple. Second Focal Plane scopes work just as well as first focal plane scopes. In some of those First Focal Plane scopes when you adjust the magnification you can obscure the target or loose your mil dots in the back ground. I prefer my second focal plane scope because it allows me a wider field of view to range my shots so I can take other measurements like wind value and comparison measurements. It is all about preference. If you don’t know how to use the equipment don’t make judgements. First focal planes are nice and can make your job easier, but not having your sights on the first plane doesn’t make your scope useless. Do more research please.

    • If you want to give yourself an extra fractional division to do, another source of measurement error, then maybe SFP scopes are no big deal. I respect and fear Murphy. He already has too many opportunities to mess with me when I’m behind a rifle. FFP eliminates one.

  6. Forget you ever learned that 27.78 junk! The math is much easier, whichever units you use, with this equation:

    (Known Height of Target x 1000) / (Height of Target in Mils) = Distance To Target

    For a 2-meter high target (a really tall guy) that appears to be 10 mils tall in the scope, that range works out to 200 meters.

    (2 meters x 1000)/10 mils=200 meters distant

    It also works for feet, inches or fathoms. What about a 5.8 foot target (5’10” or so) who appears to be 5 mils tall in the scope?

    (5.8 feet x 1000)/5 mils=1160 feet.

    I’ve been trying to use this in my own Leupold scope testing. The hard part is finding guys 2 meters tall and getting them walk a few blocks down the street and hold still while I scope them…

    • That equation is similar to the one I was taught except it was
      (Height in inches x 27.77) / Height in Mills = Range in yards

      I can see how the Height X 1,000 would be much easier to calculate in your head.

      I have heard of several other calculation methods. But one you find one you like just practice it.

    • You beat me to it. If you want to do it in your head, just remember that 1 mil in size = 1000 of whatever that size is. So a 6′ man, 3 mils in apparent height 6/3*1000 = 2000 ft’ = 666 yards. Or say you’re ranging an 18″ wheel on a vehicle, and it’s 1 mil high. 1.5′ * 1000 = 1500 ft’ = 500 yards.

      Of course it’s even easier if you use centimeters. Just divide by mils and multiply by ten. 28 cm target, 3/4 mil high is 28 * 4 / 3 * 10 = 373 m.

  7. Are there mil-dot reticle variable magnification scopes where the reticle zooms along with the magnification? Otherwise the dot spacing would only be a ‘mil’ at some particular magnification, yes?

    • If the reticle is in the first focal plane, it will grow and shrink as you zoom in and out. If it’s in the second, then yes, the dot is a mil only at a set magnification. You can still use the mil dots at different magnifications even with second focal plane reticles, but you have to know what they mean that the magnification you’re at, e.g. at a certain magnification a dot might be two mils and you’d adjust the math accordingly.

  8. Dillon Reloading (catalog – The Blue Press) sells a “Mil-Dot Master” stiffish coated cardboard handheld, nonpowered mil-dot “computer” to assist in the calculations. Around $30. Sort of a slide rule for mil-dot (if any of you young whippersnappers know what a slide rule is). Hey, when the US electric power grid and all of the computers are fried by a major solar flare (as in 1859) or an Iranian EMP attack, some of us still have our college sliderules WITH instructions and geek belt case. Go low tech!

    • My high school math teacher got mad at me because, when he told us no calculators, I showed up with a real nice pocket slide rule my father gave me. So, yeah, some of us remember.

  9. My head hurts. First, no more hot chick-pictures standing above the average of 5″4″ for copyright reasons. Now this? If you have to shoot someone at 200 yds and you aren’t in Afghanistan, turn and run.

    I suppose there is hunting though…

  10. Call me old school, or just old, but I never estimated ranges using math. Why? Because I HATE doing math, that’s why. Hate is not too strong a word. In fact, I became a lawyer so I’d never have to do math, which made for interesting billings. I think that charts and tables and slide rules (‘memba them?) and hand-held ballistic calculators make excellent targets and should be shot on sight. I guess that makes me a curmudgeon. Mea culpa.

    I use two workarounds to avoid the math trap. First, anyone who is serious about golf has a handheld range finder. I hate golf as much as I hate math, but the rangefinder is great up to a few hundred yards. It fits in a pcket, weighs a couple of ounces, runs on regular batteries and it’s waaaaaaaaaaaaaay cheaper than a scope with a built-in laser rangefinder. No math required.

    Second, high power scopes often have objective lenses that need to be focused. Those lenses have hash marks indicating distances up to 600 yards on some scopes, although some top out at 300. It’s not as precise as a laser rangefinder, but it’s a reasonably accurate way of estimating distances.

    Now I have to go shoot something. Just thinking about math is making me twitchy.

  12. Keep an eye out for an upcoming Gear Review of the “Mid-Dot Master”, which makes easy work of range estimation, bullet drop, and incline adjustment!

  13. SFP scopes are useless for range estimation.

    1. Who knows what magnification you’re actually at? Is it really 10X or is it 9.5X, and is it the same as it was the last time it was set there? I hope the numbers in my shot book don’t stay the same size when I get closer to them.

    2. Do I really have to use a different formula for each power setting? I sure am glad I have a FFP scope.

    I can’t even believe I had to just say that.

  14. I was just looking around google and found this website, as I have been mulling over a few things about mil dot scopes I thought I’d put up an idea here and see what folks thought of it.

    The calculations are straightforward for estimating distances with a mil dot scope. I have a variable power mil dot, the pattern is the same size at any power, but it is only accurate (1 mil = 1 yard at 1000 yards) at the highest power. I also have another one that the mil dots change with the power setting and I suspect that is accurate at any magnification ( first v second plane) but I am NOT talking about that one, just the one that the pattern stays the same.

    Correct me if I am wrong, but on 12 (high) power, the scope will say that a 2 yard tall man is 2 mils high at 1000 yard. It would follow the same man is 4 mils high at 500 yards, and this is the basis for mil dot ranging using the traditional formula.

    HOWEVER, my 2 yard tall man will also be 2 mills high at 500 yards if I dial my scope down to 6 power. At 4 power, that same man will be 2 mils high at 333 years, and at 3 he will be at 250. I can easily calculate that to make him 2 mills high at 300 yards, my scope will be at 3.6 power. I am sure you can see where I am going with this.

    Say there is a vehicle parked near my target and I know the distance between the center of the front and rear tire is 3 yards. To range it I would adjust the power on my scope until there was a mil dot in the center of the front wheel, and three dots down the line was in the center of the rear wheel (for demonstration purposes we will agree the vehicle is exactly perpendicular to my line of sight). I then just read the magnification on the scope and see that about a quarter of the way between 7 and 8. My estimation of the range is 600 yards based on the magnification needed to make each mil dot 1 yard apart at the target.

    Any thoughts?

    Bob

Comments are closed.